Polyrhons
2017/07/05
The rhombic dodecahedron is a convex polyhedron with 12 congruent rhombic faces. It can be used to tessalate three-dimensional space.
If we connect two or more of them at their faces we get poly-rhombic-dodecahedrons or for short polyrhons. There are 28
tetrarhons and I was asked by Jared McComb to look for a construction of two tetrahedrons made with these pieces.
I found a solution for this problem and it's even possible to use the 14 planar tetrarhons for the first tetrahedron and the 14 nonplanar pieces
for the second one. Carl Hoff created the physical pieces, showed them at
YouTube and you can get his planar pieces at
Shapeways.
Some time later I assembled a low cost 3-D-printer and produced obj-files for the pieces with my computer program. The files are
available at Thingiverse. Here are the printed physical pieces.
Among the planar pieces there are three pairs of mirrored pieces and among the nonplanar pieces you can see
four symmetric pieces and five mirror twins.
Since my computer program doesn't check for wrapped pieces, which don't fit as physical pieces, I checked some of the solutions
by putting the real pieces together. Pieces, which slide down in the physical construction, are another problem.
A rhombic dodecahedron has six corners of order four, where four edges meet. A construction with such corners at the
bottom often crashed. But when it is turned around an horizontal axis by 90 degree the rhombic faces are at the bottom and
the construction may be stable.
Due to the kind of layers three different presentations of computer solutions are used:
-
If corners of order four are at the bottom, each layer makes a square grid.
-
If rhombs are at the bottom, the layers are hexagons in a rectangular grid.
-
If corners, where three edges meet, are at the bottom, each layer makes an hexagonal grid.
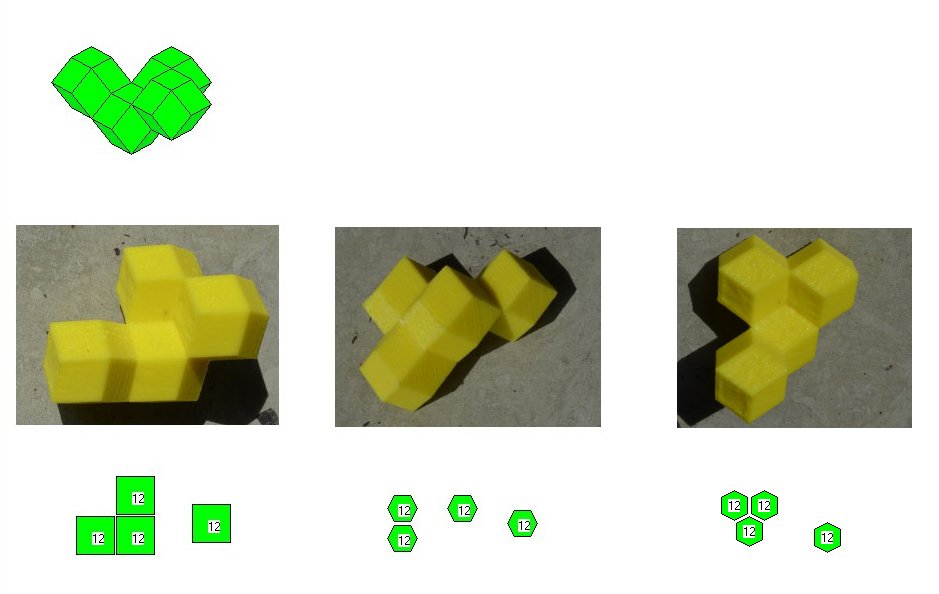
For the following sets I found some constructions. Click the sets to see pictures and solutions.
Home