Polyhalfhexes

2004/04/29

Here are some concrete paving-stones. The half hexagons are used to get straight borders for the paving but you can also join these pieces at their sides to get a lot of different forms. Some constructions are shown for the following sets:

Three halfhexes - 15 two-sided pieces
Three halfhexes - 25 one-sided pieces
Four halfhexes - 82 two-sided pieces

You can also find nice symmetric figures with these sets on Andrew Clarke's site.

Two-sided Trihalfhexes

There are 15 different pieces. We can look for 4-fold replicas of each piece, if we use the replicated piece twice. The solutions are shown as similar hole figures.

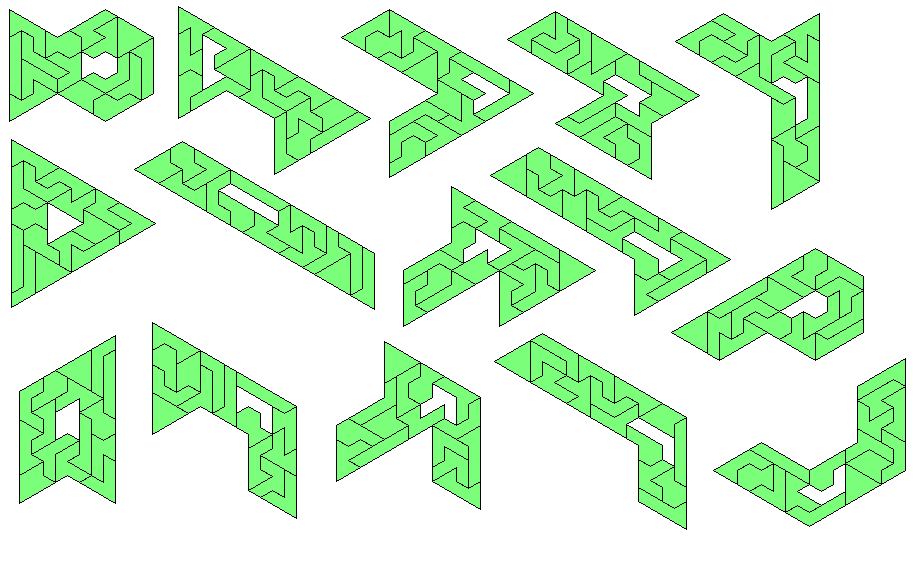
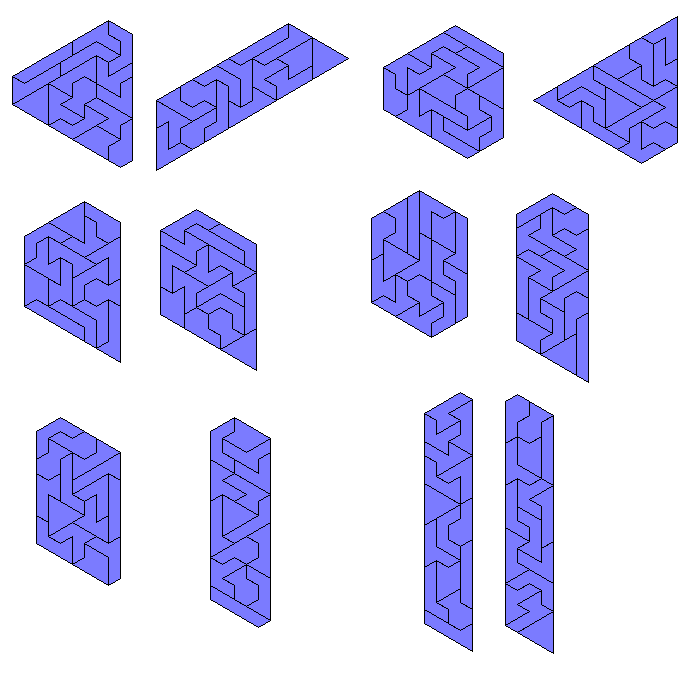
If you don't like holes in your constructions, you can ask for possible convex polygons. But there are only four symmetric and eight non-symmetric convex polygons. I hope I didn't miss any.

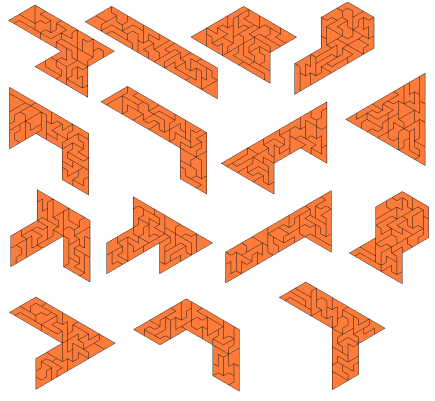
One-sided Trihalfhexes

We have 25 different pieces. If you want to create the pieces with my solver, you will get 26 pieces. There are two triangles in the set, which are different only if the grid structure is shown. Therefore I decided to remove one of them from the set. You can get the pieces if you take the two-sided set and add the 10 mirror pieces of the nonsymmetric elements.
25 pieces allow for 5-fold replicas of each piece and you can see all solutions. For the mirror pieces just reflect the constructions.

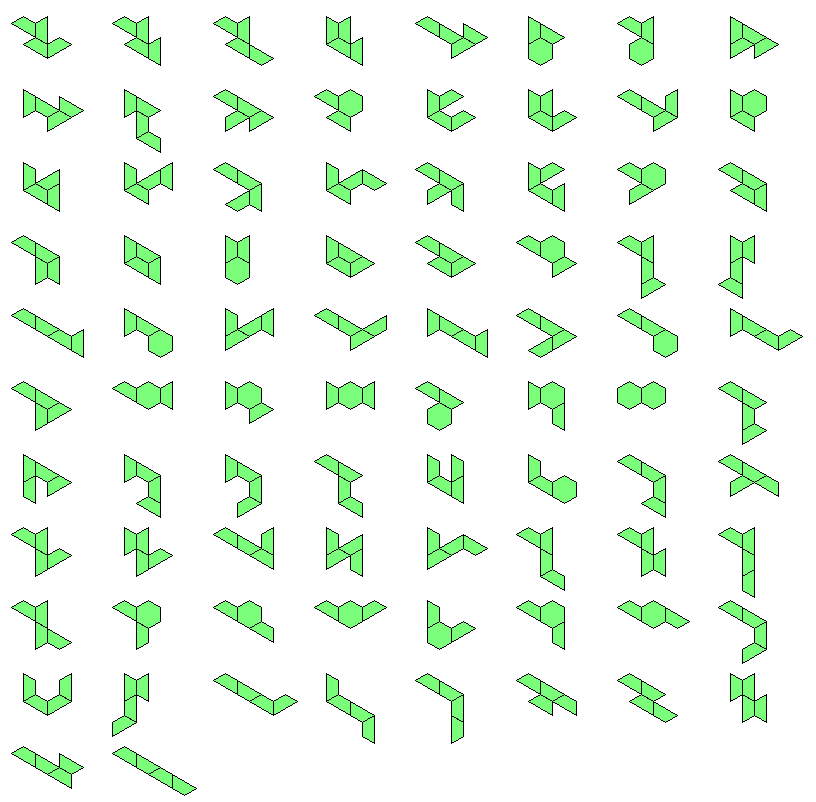
Two-sided Tetrahalfhexes

Here are the 82 pieces.

All 9-fold replicas of the pieces without using the replicated piece are possible. The computer can find the solutions rather fast. But it is important to split the figures into two parts and to choose the right sequences to fill the parts. Furthermore you have to save good pieces for the end of the game. Look at the replicas of the symmetric pieces to find the separating lines between the parts.

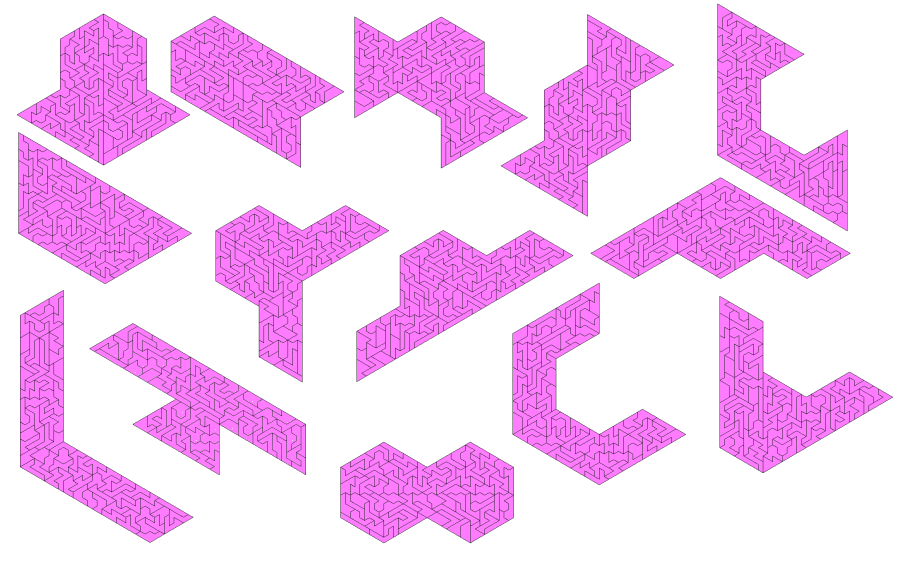
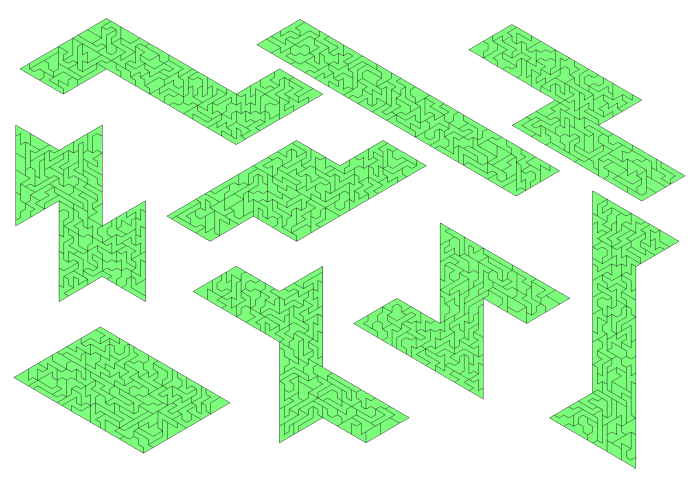The replicas of the other pieces are here.

A size-14-hexagon with a hole shaped as a 4-fold copy of each piece should be possible, too. I didn't want to enter all 82 figures and tried only one. Perhaps I'll write a program later to do the job.

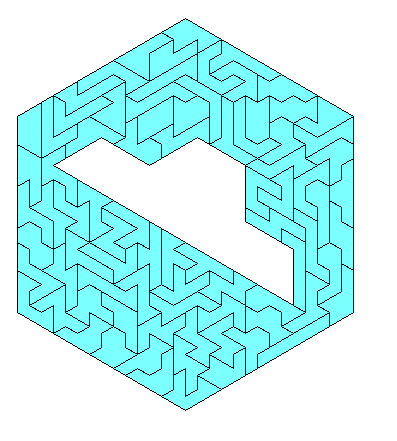
(Two weeks later I started with the other hole replicas and finished the work in a couple of days without writing a special program. You can find the constructions for all pieces here.)

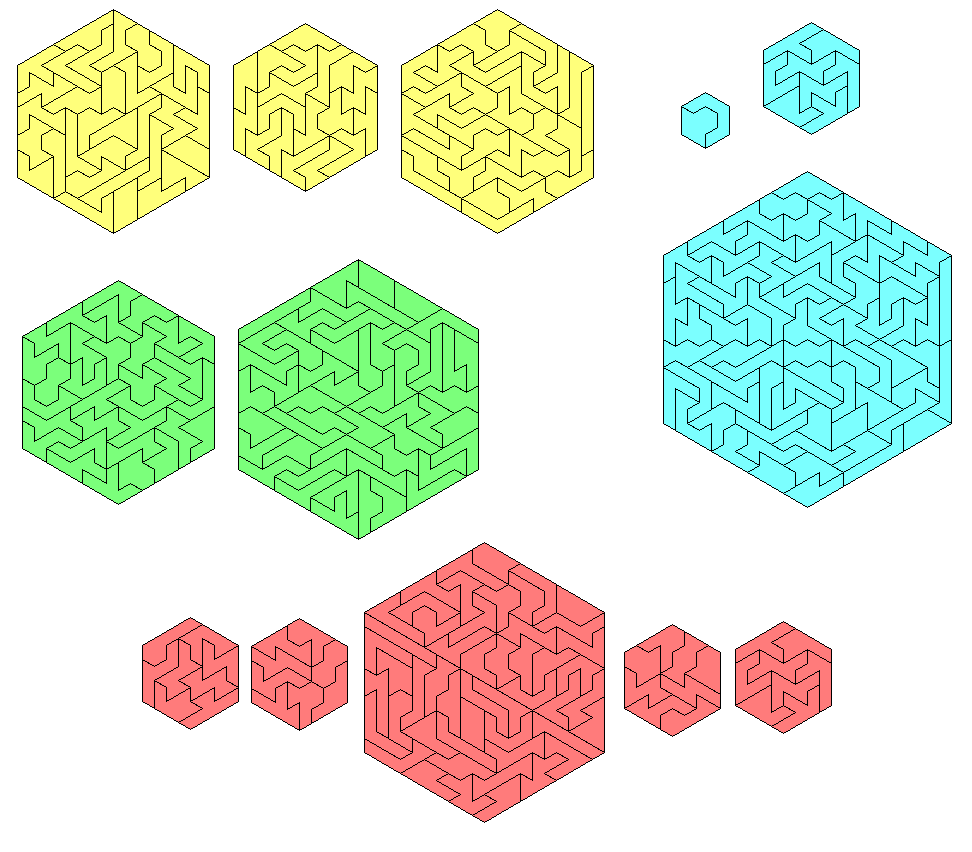
With each piece consisting of 4x3=12 small triangles n^2/2 pieces can be used to make a hexagon of size n. Some constructions of multiple hexagons are shown. What is the maximum number of hexagons which can be obtained?

Home